Representation Theory

• B1.6

  Representation Theory

  Part II, 2001

  Compute the character table of $A_{5}$ (begin by listing the conjugacy classes and their orders).

  [It is not enough to write down the result; you must justify your answer.]

  comment

• B2.6

  Representation Theory

  Part II, 2001

  (i) Let $G$ be a group, and $X$ and $Y$ finite $G$-sets. Define the permutation representation $\mathbf{C}[X]$ and compute its character. Show that

  $$\operatorname{dim}_{\operatorname{Hom}_{G}}(\mathbf{C}[X], \mathbf{C}[Y])$$

  is equal to the number of $G$-orbits in $X \times Y$.

  (ii) Let $G=S_{n}(n \geqslant 4), X=\{1, \ldots, n\}$, and

  $$Z=\{\{i, j\} \subseteq X \mid i \neq j\}$$

  be the set of 2 -element subsets of $X$. Decompose $\mathbf{C}[Z]$ into irreducibles, and determine the dimension of each irreducible constituent.

  comment

• B3.5

  Representation Theory

  Part II, 2001

  Let $G=S U_{2}$, and $V_{n}$ be the vector space of homogeneous polynomials of degree $n$ in the variables $x$ and $y$.

  (i) Define the action of $G$ on $V_{n}$, and prove that $V_{n}$ is an irreducible representation of $G$.

  (ii) Decompose $V_{4} \otimes V_{3}$ into irreducible representations of $S U_{2}$. Briefly justify your answer.

  (iii) $S U_{2}$ acts on the vector space $M_{3}(\mathbf{C})$ of complex $3 \times 3$ matrices via

  $$\rho\left(\begin{array}{ll} a & b \\ c & d \end{array}\right) \cdot X=\left(\begin{array}{lll} a & b & 0 \\ c & d & 0 \\ 0 & 0 & 1 \end{array}\right) X\left(\begin{array}{lll} a & b & 0 \\ c & d & 0 \\ 0 & 0 & 1 \end{array}\right)^{-1}, \quad X \in M_{3}(\mathbf{C}) .$$

  Decompose this representation into irreducible representations.

  comment

• B4.2

  Representation Theory

  Part II, 2001

  Let $G$ be the Heisenberg group of order $p^{3}$. This is the subgroup

  $$G=\left\{\left(\begin{array}{ccc} 1 & a & x \\ 0 & 1 & b \\ 0 & 0 & 1 \end{array}\right) \mid a, b, x \in \mathbf{F}_{p}\right\}$$

  of $3 \times 3$ matrices over the finite field $\mathbf{F}_{p}$ ( $p$ prime). Let $H$ be the subgroup of $G$ of such matrices with $a=0$.

  (i) Find all one dimensional representations of $G$.

  [You may assume without proof that $[G, G]$ is equal to the set of matrices in $G$ with $a=b=0 .]$

  (ii) Let $\psi: \mathbf{F}_{p}=\mathbf{Z} / p \mathbf{Z} \longrightarrow \mathbf{C}^{*}$ be a non-trivial one dimensional representation of $\mathbf{F}_{p}$, and define a one dimensional representation $\rho$ of $H$ by

  $$\rho\left(\begin{array}{lll} 1 & 0 & x \\ 0 & 1 & b \\ 0 & 0 & 1 \end{array}\right)=\psi(x)$$

  Show that $V_{\psi}=\operatorname{Ind}_{H}^{G}(\rho)$ is irreducible.

  (iii) List all the irreducible representations of $G$ and explain why your list is complete.

  comment

• B1.6

  Representation Theory

  Part II, 2002

  Construct the character table of the symmetric group $S_{5}$, explaining the steps in your construction.

  Use the character table to show that the alternating group $A_{5}$ is the only non-trivial normal subgroup of $S_{5}$.

  comment

• B2.6

  Representation Theory

  Part II, 2002

  State and prove Schur's Lemma. Deduce that the centre of a finite group $G$ with a faithful irreducible complex representation $\rho$ is cyclic and that $Z(\rho(G))$ consists of scalar transformations.

  Let $G$ be the subgroup of order 18 of the symmetric group $S_{6}$ given by

  $$G=\langle(123),(456),(23)(56)\rangle$$

  Show that $G$ has a normal subgroup of order 9 and four normal subgroups of order 3 . By considering quotients, show that $G$ has two representations of dimension 1 and four inequivalent irreducible representations of degree 2 . Deduce that $G$ has no faithful irreducible complex representations.

  Show finally that if $G$ is a finite group with trivial centre and $H$ is a subgroup of $G$ with non-trivial centre, then any faithful representation of $G$ is reducible on restriction to $H$.

  comment

• B3.5

  Representation Theory

  Part II, 2002

  Let $G$ be a finite group acting on a finite set $X$. Define the permutation representation $(\rho, \mathbb{C}[X])$ of $G$ and compute its character $\pi_{X}$. Prove that $\left\langle\pi_{X}, 1_{G}\right\rangle_{G}$ equals the number of orbits of $G$ on $X$. If $G$ acts also on the finite set $Y$, with character $\pi_{Y}$, show that $\left\langle\pi_{X}, \pi_{Y}\right\rangle_{G}$ equals the number of orbits of $G$ on $X \times Y$.

  Now let $G$ be the symmetric group $S_{n}$ acting naturally on the set $X=\{1, \ldots, n\}$, and let $X_{r}$ be the set of all $r$-element subsets of $X$. Let $\pi_{r}$ be the permutation character of $G$ on $X_{r}$. Prove that

  $$\left\langle\pi_{k}, \pi_{\ell}\right\rangle_{G}=\ell+1 \text { for } 0 \leqslant \ell \leqslant k \leqslant n / 2$$

  Deduce that the class functions

  $$\chi_{r}=\pi_{r}-\pi_{r-1}$$

  are irreducible characters of $S_{n}$, for $1 \leqslant r \leqslant n / 2$.

  comment

• B4.2

  Representation Theory

  Part II, 2002

  Write an essay on the representation theory of $S U_{2}$.

  comment

• B1.6

  Representation Theory

  Part II, 2003

  Define the inner product $\langle\varphi, \psi\rangle$ of two class functions from the finite group $G$ into the complex numbers. Prove that characters of the irreducible representations of $G$ form an orthonormal basis for the space of class functions.

  Consider the representation $\pi: S_{n} \rightarrow G L_{n}(\mathbb{C})$ of the symmetric group $S_{n}$ by permutation matrices. Show that $\pi$ splits as a direct sum $1 \oplus \rho$ where 1 denotes the trivial representation. Is the $(n-1)$-dimensional representation $\rho$ irreducible?

  comment

• B2.6

  Representation Theory

  Part II, 2003

  Let $V_{n}$ be the space of homogeneous polynomials of degree $n$ in two variables $z_{1}$ and $z_{2}$. Define a left action of $G=S U_{2}$ on the space of polynomials by setting

  $$(g P) z=P(z g),$$

  where $P \in \mathbb{C}\left[z_{1}, z_{2}\right], \quad g=\left(\begin{array}{cc}a & b \\ c & d\end{array}\right), \quad z=\left(z_{1}, z_{2}\right) \quad$ and $\quad z g=\left(a z_{1}+c z_{2}, b z_{1}+d z_{2}\right)$.

  Show that

  (a) the representations $V_{n}$ are irreducible,

  (b) the representations $V_{n}$ exhaust the irreducible representations of $S U_{2}$, and

  

  comment

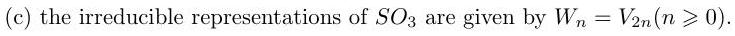
• B3.5

  Representation Theory

  Part II, 2003

  If $\rho_{1}: G_{1} \rightarrow G L\left(V_{1}\right)$ and $\rho_{2}: G_{2} \rightarrow G L\left(V_{2}\right)$ are representations of the finite groups $G_{1}$ and $G_{2}$ respectively, define the tensor product $\rho_{1} \otimes \rho_{2}$ as a representation of the group $G_{1} \times G_{2}$ and show that its character is given by

  $$\chi_{\rho_{1} \otimes \rho_{2}}\left(g_{1}, g_{2}\right)=\chi_{\rho_{1}}\left(g_{1}\right) \chi_{\rho_{2}}\left(g_{2}\right)$$

  Prove that

  (a) if $\rho_{1}$ and $\rho_{2}$ are irreducible, then $\rho_{1} \otimes \rho_{2}$ is an irreducible representation of $G_{1} \times G_{2}$;

  (b) each irreducible representation of $G_{1} \times G_{2}$ is equivalent to a representation $\rho_{1} \otimes \rho_{2}$ where each $\rho_{i}$ is irreducible $(i=1,2) .$

  Is every representation of $G_{1} \times G_{2}$ the tensor product of a representation of $G_{1}$ and a representation of $G_{2}$ ?

  comment

• B4.2

  Representation Theory

  Part II, 2003

  Assume that the group $S L_{2}\left(\mathbb{F}_{3}\right)$ of $2 \times 2$ matrices of determinant 1 with entries from the field $\mathbb{F}_{3}$ has presentation

  $$\left\langle X, P, Q: X^{3}=P^{4}=1, P^{2}=Q^{2}, P Q P^{-1}=Q^{-1}, X P X^{-1}=Q, X Q X^{-1}=P Q\right\rangle$$

  Show that the subgroup generated by $P^{2}$ is central and that the quotient group can be identified with the alternating group $A_{4}$. Assuming further that $S L_{2}\left(\mathbb{F}_{3}\right)$ has seven conjugacy classes find the character table.

  Is it true that every irreducible character is induced up from the character of a 1-dimensional representation of some subgroup?

  [Hint: You may find it useful to note that $S L_{2}\left(\mathbb{F}_{3}\right)$ may be regarded as a subgroup of $S U_{2}$, providing a faithful 2-dimensional representation; the subgroup generated by $P$ and $Q$ is the quaternion group of order 8 , acting irreducibly.]

  comment

• B1.6

  Representation Theory

  Part II, 2004

  (a) Show that every irreducible complex representation of an abelian group is onedimensional.

  (b) Show, by example, that the analogue of (a) fails for real representations.

  (c) Let the cyclic group of order $n$ act on $\mathbb{C}^{n}$ by cyclic permutation of the standard basis vectors. Decompose this representation explicitly into irreducibles.

  comment

• B2.6

  Representation Theory

  Part II, 2004

  Let $H$ be a group with three generators $c, g, h$ and relations $c^{p}=g^{p}=h^{p}=1$, $c g=g c, c h=h c$ and $g h=c h g$ where $p$ is a prime number.

  (a) Show that $|H|=p^{3}$. Show that the conjugacy classes of $H$ are the singletons $\{1\},\{c\}, \ldots,\left\{c^{p-1}\right\}$ and the sets $\left\{g^{m} h^{n}, c g^{m} h^{n}, \ldots, c^{p-1} g^{m} h^{n}\right\}$, as $m, n$ range from 0 to $p-1$, but $(m, n) \neq(0,0)$.

  (b) Find $p^{2}$ 1-dimensional representations of $H$.

  (c) Let $\omega \neq 1$ be a $p$ th root of unity. Show that the following defines an irreducible representation of $H$ on $\mathbb{C}^{p}$ :

  $$\begin{gathered} \rho(c)=\omega \mathrm{Id}, \\ \rho(g) \mathbf{e}_{k}=\omega^{k} \mathbf{e}_{k}, \\ \rho(h) \mathbf{e}_{p}=\mathbf{e}_{1} \text { and } \rho(h) \mathbf{e}_{k}=\mathbf{e}_{k+1} \text { if } k<p \end{gathered}$$

  where the $\mathbf{e}_{k}$ are the standard basis vectors of $\mathbb{C}^{p}$.

  (d) Show that (b) and (c) cover all irreducible isomorphism classes.

  comment

• B3.5

  Representation Theory

  Part II, 2004

  Compute the character table for the group $A_{5}$ of even permutations of five elements. You may wish to follow the steps below.

  (a) List the conjugacy classes in $A_{5}$ and their orders.

  (b) $A_{5}$ acts on $\mathbb{C}^{5}$ by permuting the standard basis vectors. Show that $\mathbb{C}^{5}$ splits as $\mathbb{C} \oplus V$, where $\mathbb{C}$ is the trivial 1-dimensional representation and $V$ is irreducible.

  (c) By using the formula for the character of the symmetric square $S^{2} V$,

  $$\chi_{S^{2} V}(g)=\frac{1}{2}\left[\chi_{V}(g)^{2}+\chi_{V}\left(g^{2}\right)\right]$$

  decompose $S^{2} V$ to produce a 5-dimensional, irreducible representation, and find its character.

  (d) Show that the exterior square $\Lambda^{2} V$ decomposes into two distinct irreducibles and compute their characters, to complete the character table of $A_{5}$.

  [Hint: You can save yourself some computational effort if you can explain why the automorphism of $A_{5}$, defined by conjugation by a transposition in $S_{5}$, must swap the two summands of $\Lambda^{2} V$.]

  comment

• B4.2

  Representation Theory

  Part II, 2004

  Write an essay on the finite-dimensional representations of $S U_{2}$, including a proof of their complete reducibility, and a description of the irreducible representations and the decomposition of their tensor products.

  comment

• 1.II.19G

  Representation Theory

  Part II, 2005

  Let the finite group $G$ act on finite sets $X$ and $Y$, and denote by $\mathbb{C}[X], \mathbb{C}[Y]$ the associated permutation representations on the spaces of complex functions on $X$ and $Y$. Call their characters $\chi_{X}$ and $\chi_{Y}$.

  (i) Show that the inner product $\left\langle\chi_{X} \mid \chi_{Y}\right\rangle$ is the number of orbits for the diagonal action of $G$ on $X \times Y$.

  (ii) Assume that $|X|>1$, and let $S \subset \mathbb{C}[X]$ be the subspace of those functions whose values sum to zero. By considering $\left\|\chi_{X}\right\|^{2}$, show that $S$ is irreducible if and only if the $G$-action on $X$ is doubly transitive: this means that for any two pairs $\left(x_{1}, x_{2}\right)$ and $\left(x_{1}^{\prime}, x_{2}^{\prime}\right)$ of points in $X$ with $x_{1} \neq x_{2}$ and $x_{1}^{\prime} \neq x_{2}^{\prime}$, there exists some $g \in G$ with $g x_{1}=x_{1}^{\prime}$ and $g x_{2}=x_{2}^{\prime}$.

  (iii) Let now $G=S_{n}$ acting on the set $X=\{1,2, \ldots, n\}$. Call $Y$ the set of 2element subsets of $X$, with the natural action of $S_{n}$. If $n \geqslant 4$, show that $\mathbb{C}[Y]$ decomposes under $S_{n}$ into three irreducible representations, one of which is the trivial representation and another of which is $S$. What happens when $n=3$ ?

  [Hint: Consider $\left\langle 1 \mid \chi_{Y}\right\rangle,\left\langle\chi_{X} \mid \chi_{Y}\right\rangle$ and $\left\|\chi_{Y}\right\|^{2}$.]

  comment

• 2.II.19G

  Representation Theory

  Part II, 2005

  Let $G$ be a finite group and $\left\{\chi_{i}\right\}$ the set of its irreducible characters. Also choose representatives $g_{j}$ for the conjugacy classes, and denote by $Z\left(g_{j}\right)$ their centralisers.

  (i) State the orthogonality and completeness relations for the $\chi_{k}$.

  (ii) Using Part (i), or otherwise, show that

  $$\sum_{i} \overline{\chi_{i}\left(g_{j}\right)} \cdot \chi_{i}\left(g_{k}\right)=\delta_{j k} \cdot\left|Z\left(g_{j}\right)\right|$$

  (iii) Let $A$ be the matrix with $A_{i j}=\chi_{i}\left(g_{j}\right)$. Prove that

  $$|\operatorname{det} A|^{2}=\prod_{j}\left|Z\left(g_{j}\right)\right|$$

  (iv) Show that $\operatorname{det} A$ is either real or purely imaginary, explaining when each situation occurs.

  [Hint for (iv): Consider the effect of complex conjugation on the rows of the matrix A.]

  comment

• 3.II.19G

  Representation Theory

  Part II, 2005

  Let $G$ be the group with 21 elements generated by $a$ and $b$, subject to the relations $a^{7}=b^{3}=1$ and $b a=a^{2} b .$

  (i) Find the conjugacy classes of $G$.

  (ii) Find three non-isomorphic one-dimensional representations of $G$.

  (iii) For a subgroup $H$ of a finite group $K$, write down (without proof) the formula for the character of the $K$-representation induced from a representation of $H$.

  (iv) By applying Part (iii) to the case when $H$ is the subgroup $\langle a\rangle$ of $K=G$, find the remaining irreducible characters of $G$.

  comment

• 4.II.19G

  Representation Theory

  Part II, 2005

  (i) State and prove the Weyl integration formula for $S U(2)$.

  (ii) Determine the characters of the symmetric powers of the standard 2-dimensional representation of $S U(2)$ and prove that they are irreducible.

  [Any general theorems from the course may be used.]

  comment

• 1.II.19F

  Representation Theory

  Part II, 2006

  (a) Let $G$ be a finite group and $X$ a finite set on which $G$ acts. Define the permutation representation $\mathbb{C}[X]$ and compute its character.

  (b) Let $G$ and $U$ be the following subgroups of $\mathrm{GL}_{2}\left(\mathbb{F}_{p}\right)$, where $p$ is a prime,

  $$G=\left\{\left(\begin{array}{cc} a & b \\ 0 & 1 \end{array}\right) \mid a \in \mathbb{F}_{p}^{\times}, b \in \mathbb{F}_{p}\right\}, \quad U=\left\{\left(\begin{array}{ll} 1 & b \\ 0 & 1 \end{array}\right) \mid b \in \mathbb{F}_{p}\right\}$$

  (i) Decompose $\mathbb{C}[G / U]$ into irreducible representations.

  (ii) Let $\psi: U \rightarrow \mathbb{C}^{\times}$be a non-trivial, one-dimensional representation. Determine the character of the induced representation $\operatorname{Ind}_{U}^{G} \psi$, and decompose $\operatorname{Ind}_{U}^{G} \psi$ into irreducible representations.

  (iii) List all of the irreducible representations of $G$ and show that your list is complete.

  comment

• 2.II.19F

  Representation Theory

  Part II, 2006

  (a) Let $G$ be $S_{4}$, the symmetric group on four letters. Determine the character table of $G$.

  [Begin by listing the conjugacy classes and their orders.]

  (b) For each irreducible representation $V$ of $G=S_{4}$, decompose $\operatorname{Res}_{A_{4}}^{S_{4}}(V)$ into irreducible representations. You must justify your answer.

  comment

• 3.II.19F

  Representation Theory

  Part II, 2006

  (a) Let $G=\mathrm{SU}_{2}$, and let $V_{n}$ be the space of homogeneous polynomials of degree $n$ in the variables $x$ and $y$. Thus $\operatorname{dim} V_{n}=n+1$. Define the action of $G$ on $V_{n}$ and show that $V_{n}$ is an irreducible representation of $G$.

  (b) Decompose $V_{3} \otimes V_{3}$ into irreducible representations. Decompose $\wedge^{2} V_{3}$ and $\mathrm{S}^{2} V_{3}$ into irreducible representations.

  (c) Given any representation $V$ of a group $G$, define the dual representation $V^{*}$. Show that $V_{n}^{*}$ is isomorphic to $V_{n}$ as a representation of $\mathrm{SU}_{2}$.

  [You may use any results from the lectures provided that you state them clearly.]

  comment

• 4.II.19F

  Representation Theory

  Part II, 2006

  In this question, all vector spaces will be complex.

  (a) Let $A$ be a finite abelian group.

  (i) Show directly from the definitions that any irreducible representation must be one-dimensional.

  (ii) Show that $A$ has a faithful one-dimensional representation if and only if $A$ is cyclic.

  (b) Now let $G$ be an arbitrary finite group and suppose that the centre of $G$ is nontrivial. Write $Z=\{z \in G \mid z g=g z \quad \forall g \in G\}$ for this centre.

  (i) Let $W$ be an irreducible representation of $G$. Show that $\operatorname{Res}_{Z}^{G} W=\operatorname{dim} W \cdot \chi$, where $\chi$ is an irreducible representation of $Z$.

  (ii) Show that every irreducible representation of $Z$ occurs in this way.

  (iii) Suppose that $Z$ is not a cyclic group. Show that there does not exist an irreducible representation $W$ of $G$ such that every irreducible representation $V$ occurs as a summand of $W^{\otimes n}$ for some $n$.

  comment

• 1.II.19H

  Representation Theory

  Part II, 2007

  A finite group $G$ has seven conjugacy classes $C_{1}=\{e\}, C_{2}, \ldots, C_{7}$ and the values of five of its irreducible characters are given in the following table.

  $\begin{array}{rrrrrrr}C_{1} & C_{2} & C_{3} & C_{4} & C_{5} & C_{6} & C_{7} \\ 1 & 1 & 1 & 1 & 1 & 1 & 1 \\ 1 & 1 & 1 & 1 & -1 & -1 & -1 \\ 4 & 0 & 1 & -1 & 2 & -1 & 0 \\ 4 & 0 & 1 & -1 & -2 & 1 & 0 \\ 5 & 1 & -1 & 0 & 1 & 1 & -1\end{array}$

  Calculate the number of elements in the various conjugacy classes and complete the character table.

  [You may not identify $G$ with any known group, unless you justify doing so.]

  comment

• 2.II.19H

  Representation Theory

  Part II, 2007

  Let $G$ be a finite group and let $Z$ be its centre. Show that if $\rho$ is a complex irreducible representation of $G$, assumed to be faithful (that is, the kernel of $\rho$ is trivial), then $Z$ is cyclic.

  Now assume that $G$ is a p-group (that is, the order of $G$ is a power of the prime $p)$, and assume that $Z$ is cyclic. If $\rho$ is a faithful representation of $G$, show that some irreducible component of $\rho$ is faithful.

  [You may use without proof the fact that, since $G$ is a p-group, $Z$ is non-trivial and any non-trivial normal subgroup of $G$ intersects $Z$ non-trivially.]

  Deduce that a finite $p$-group has a faithful irreducible representation if and only if its centre is cyclic.

  comment

• 3.II.19H

  Representation Theory

  Part II, 2007

  Let $G$ be a finite group with a permutation action on the set $X$. Describe the corresponding permutation character $\pi_{X}$. Show that the multiplicity in $\pi_{X}$ of the principal character $1_{G}$ equals the number of orbits of $G$ on $X$.

  Assume that $G$ is transitive on $X$, with $|X|>1$. Show that $G$ contains an element $g$ which is fixed-point-free on $X$, that is, $g \alpha \neq \alpha$ for all $\alpha$ in $X$.

  Assume that $\pi_{X}=1_{G}+m \chi$, with $\chi$ an irreducible character of $G$, for some natural number $m$. Show that $m=1$.

  [You may use without proof any facts about algebraic integers, provided you state them correctly.]

  Explain how the action of $G$ on $X$ induces an action of $G$ on $X^{2}$. Assume that $G$ has $r$ orbits on $X^{2}$. If now

  $$\pi_{X}=1_{G}+m_{2} \chi_{2}+\ldots+m_{k} \chi_{k}$$

  with $1_{G}, \chi_{2}, \ldots, \chi_{k}$ distinct irreducible characters of $G$, and $m_{2}, \ldots, m_{k}$ natural numbers, show that $r=1+m_{2}^{2}+\ldots+m_{k}^{2}$. Deduce that, if $r \leqslant 5$, then $k=r$ and $m_{2}=\ldots=m_{k}=1$.

  comment

• 4.II.19H

  Representation Theory

  Part II, 2007

  Write an essay on the representation theory of $\mathrm{SU}_{2}$.

  Your answer should include a description of each irreducible representation and an explanation of how to decompose arbitrary representations into a direct sum of these.

  comment

• 1.II.19G

  Representation Theory

  Part II, 2008

  For a complex representation $V$ of a finite group $G$, define the action of $G$ on the dual representation $V^{*}$. If $\alpha$ denotes the character of $V$, compute the character $\beta$ of $V^{*}$.

  [Your formula should express $\beta(g)$ just in terms of the character $\alpha$.]

  Using your formula, how can you tell from the character whether a given representation is self-dual, that is, isomorphic to the dual representation?

  Let $V$ be an irreducible representation of $G$. Show that the trivial representation occurs as a summand of $V \otimes V$ with multiplicity either 0 or 1 . Show that it occurs once if and only if $V$ is self-dual.

  For a self-dual irreducible representation $V$, show that $V$ either has a nondegenerate $G$-invariant symmetric bilinear form or a nondegenerate $G$-invariant alternating bilinear form, but not both.

  If $V$ is an irreducible self-dual representation of odd dimension $n$, show that the corresponding homomorphism $G \rightarrow G L(n, \mathbf{C})$ is conjugate to a homomorphism into the orthogonal group $O(n, \mathbf{C})$. Here $O(n, \mathbf{C})$ means the subgroup of $G L(n, \mathbf{C})$ that preserves a nondegenerate symmetric bilinear form on $\mathbf{C}^{n}$.

  comment

• 2.II.19G

  Representation Theory

  Part II, 2008

  A finite group $G$ of order 360 has conjugacy classes $C_{1}=\{1\}, C_{2}, \ldots, C_{7}$ of sizes $1,45,40,40,90,72,72$. The values of four of its irreducible characters are given in the following table.

  $$\begin{array}{ccccccc} C_{1} & C_{2} & C_{3} & C_{4} & C_{5} & C_{6} & C_{7} \\ 5 & 1 & 2 & -1 & -1 & 0 & 0 \\ 8 & 0 & -1 & -1 & 0 & (1-\sqrt{5}) / 2 & (1+\sqrt{5}) / 2 \\ 8 & 0 & -1 & -1 & 0 & (1+\sqrt{5}) / 2 & (1-\sqrt{5}) / 2 \\ 10 & -2 & 1 & 1 & 0 & 0 & 0 \end{array}$$

  Complete the character table.

  [Hint: it will not suffice just to use orthogonality of characters.]

  Deduce that the group $G$ is simple.

  comment

• 3.II.19G

  Representation Theory

  Part II, 2008

  Let $V_{2}$ denote the irreducible representation $\operatorname{Sym}^{2}\left(\mathbb{C}^{2}\right)$ of $S U(2)$; thus $V_{2}$ has dimension 3. Compute the character of the representation $\operatorname{Sym}^{n}\left(V_{2}\right)$ of $S U(2)$ for any $n \geqslant 0$. Compute the dimension of the invariants $\operatorname{Sym}^{n}\left(V_{2}\right)^{S U(2)}$, meaning the subspace of $\operatorname{Sym}^{n}\left(V_{2}\right)$ where $S U(2)$ acts trivially.

  Hence, or otherwise, show that the ring of complex polynomials in three variables $x, y, z$ which are invariant under the action of $S O(3)$ is a polynomial ring. Find a generator for this polynomial ring.

  comment

• 4.II.19G

  Representation Theory

  Part II, 2008

  (a) Let $A$ be a normal subgroup of a finite group $G$, and let $V$ be an irreducible representation of $G$. Show that either $V$ restricted to $A$ is isotypic (a sum of copies of one irreducible representation of $A)$, or else $V$ is induced from an irreducible representation of some proper subgroup of $G$.

  (b) Using (a), show that every (complex) irreducible representation of a $p$-group is induced from a 1-dimensional representation of some subgroup.

  [You may assume that a nonabelian $p$-group $G$ has an abelian normal subgroup $A$ which is not contained in the centre of $G$.]

  comment

• Paper 4, Section II, F

  Representation Theory

  Part II, 2009

  Let $H \leqslant G$ be finite groups.

  (a) Let $\rho$ be a representation of $G$ affording the character $\chi$. Define the restriction, $\operatorname{Res}_{H}^{G} \rho$ of $\rho$ to $H$.

  Suppose $\chi$ is irreducible and suppose $\operatorname{Res}_{H}^{G} \rho$ affords the character $\chi_{H}$. Let $\psi_{1}, \ldots, \psi_{r}$ be the irreducible characters of $H$. Prove that $\chi_{H}=d_{1} \psi_{1}+\cdots+d_{r} \psi_{r}$, where the nonnegative integers $d_{1}, \ldots, d_{r}$ satisfy the inequality

  $$\sum_{i=1}^{r} d_{i}^{2} \leqslant|G: H|$$

  Prove that there is equality in (1) if and only if $\chi(g)=0$ for all elements $g$ of $G$ which lie outside $H$.

  (b) Let $\psi$ be a class function of $H$. Define the induced class function, $\operatorname{Ind}_{H}^{G} \psi$.

  State the Frobenius reciprocity theorem for class functions and deduce that if $\psi$ is a character of $H$ then $\operatorname{Ind}_{H}^{G} \psi$ is a character of $G$.

  Assuming $\psi$ is a character, identify a $G$-space affording the character $\operatorname{Ind}_{H}^{G} \psi$. Briefly justify your answer.

  (c) Let $\chi_{1}, \ldots, \chi_{k}$ be the irreducible characters of $G$ and let $\psi$ be an irreducible character of $H$. Show that the integers $e_{1}, \ldots, e_{k}$, which are given by $\operatorname{Ind}_{H}^{G}(\psi)=$ $e_{1} \chi_{1}+\cdots+e_{k} \chi_{k}$, satisfy

  $$\sum_{i=1}^{k} e_{i}^{2} \leqslant|G: H|$$

  comment

• Paper 1, Section II, F

  Representation Theory

  Part II, 2009

  Let $G$ be a finite group, and suppose $G$ acts on the finite sets $X_{1}, X_{2}$. Define the permutation representation $\rho_{X_{1}}$ corresponding to the action of $G$ on $X_{1}$, and compute its character $\pi_{X_{1}}$. State and prove "Burnside's Lemma".

  Let $G$ act on $X_{1} \times X_{2}$ via the usual diagonal action. Prove that the character inner product $\left\langle\pi_{X_{1}}, \pi_{X_{2}}\right\rangle$ is equal to the number of $G$-orbits on $X_{1} \times X_{2}$.

  Hence, or otherwise, show that the general linear group $\mathrm{GL}_{2}(q)$ of invertible $2 \times 2$ matrices over the finite field of $q$ elements has an irreducible complex representation of dimension equal to $q$.

  Let $S_{n}$ be the symmetric group acting on the set $X=\{1,2, \ldots, n\}$. Denote by $Z$ the set of all 2-element subsets $\{i, j\}(i \neq j)$ of elements of $X$, with the natural action of $S_{n}$. If $n \geqslant 4$, decompose $\pi_{Z}$ into irreducible complex representations, and determine the dimension of each irreducible constituent. What can you say when $n=3$ ?

  comment

• Paper 2, Section II, 19F

  Representation Theory

  Part II, 2009

  (i) Let $G$ be a finite group. Show that

  (1) If $\chi$ is an irreducible character of $G$ then so is its conjugate $\bar{\chi}$.

  (2) The product of any two characters of $G$ is again a character of $G$.

  (3) If $\chi$ and $\psi$ are irreducible characters of $G$ then

  $$\left\langle\chi \psi, 1_{G}\right\rangle= \begin{cases}1, & \text { if } \chi=\bar{\psi}, \\ 0, & \text { if } \chi \neq \bar{\psi} .\end{cases}$$

  (ii) If $\chi$ is a character of the finite group $G$, define $\chi_{S}$ and $\chi_{A}$. For $g \in G$ prove that

  $$\chi_{S}(g)=\frac{1}{2}\left(\chi^{2}(g)+\chi\left(g^{2}\right)\right) \quad \text { and } \chi_{A}(g)=\frac{1}{2}\left(\chi^{2}(g)-\chi\left(g^{2}\right)\right)$$

  (iii) A certain group of order 24 has precisely seven conjugacy classes with representatives $g_{1}, \ldots, g_{7}$; further, $G$ has a character $\chi$ with values as follows:

  $$\begin{array}{cccccccc} g_{i} & g_{1} & g_{2} & g_{3} & g_{4} & g_{5} & g_{6} & g_{7} \\ \left|C_{G}\left(g_{i}\right)\right| & 24 & 24 & 4 & 6 & 6 & 6 & 6 \\ \chi & 2 & -2 & 0 & -\omega^{2} & -\omega & \omega & \omega^{2} \end{array}$$

  where $\omega=e^{2 \pi i / 3}$.

  It is given that $g_{1}^{2}, g_{2}^{2}, g_{3}^{2}, g_{4}^{2}, g_{5}^{2}, g_{6}^{2}, g_{7}^{2}$ are conjugate to $g_{1}, g_{1}, g_{2}, g_{5}, g_{4}, g_{4}, g_{5}$ respectively.

  Determine $\chi_{S}$ and $\chi_{A}$, and show that both are irreducible.

  comment

• Paper 3, Section II, F

  Representation Theory

  Part II, 2009

  Let $G=\mathrm{SU}(2)$. Let $V_{n}$ be the complex vector space of homogeneous polynomials of degree $n$ in two variables $z_{1}, z_{2}$. Define the usual left action of $G$ on $V_{n}$ and denote by $\rho_{n}: G \rightarrow \operatorname{GL}\left(V_{n}\right)$ the representation induced by this action. Describe the character $\chi_{n}$ afforded by $\rho_{n}$.

  Quoting carefully any results you need, show that

  (i) The representation $\rho_{n}$ has dimension $n+1$ and is irreducible for $n \in \mathbb{Z}_{\geqslant 0}$;

  (ii) Every finite-dimensional continuous irreducible representation of $G$ is one of the $\rho_{n}$;

  (iii) $V_{n}$ is isomorphic to its dual $V_{n}^{*}$.

  comment

• Paper 1, Section II, F

  Representation Theory

  Part II, 2010

  (i) Let $N$ be a normal subgroup of the finite group $G$. Without giving detailed proofs, define the process of lifting characters from $G / N$ to $G$. State also the orthogonality relations for $G$.

  (ii) Let $a, b$ be the following two permutations in $S_{12}$,

  and let $G=\langle a, b\rangle$, a subgroup of $S_{12}$. Prove that $G$ is a group of order 12 and list the conjugacy classes of $G$. By identifying a normal subgroup of $G$ of index 4 and lifting irreducible characters, calculate all the linear characters of $G$. Calculate the complete character table of $G$. By considering 6 th roots of unity, find explicit matrix representations affording the non-linear characters of $G$.

  comment

• Paper 2, Section II, F

  Representation Theory

  Part II, 2010

  Define the concepts of induction and restriction of characters. State and prove the Frobenius Reciprocity Theorem.

  Let $H$ be a subgroup of $G$ and let $g \in G$. We write $\mathcal{C}(g)$ for the conjugacy class of $g$ in $G$, and write $C_{G}(g)$ for the centraliser of $g$ in $G$. Suppose that $H \cap \mathcal{C}(g)$ breaks up into $m$ conjugacy classes of $H$, with representatives $x_{1}, x_{2}, \ldots, x_{m}$.

  Let $\psi$ be a character of $H$. Writing $\operatorname{Ind}_{H}^{G}(\psi)$ for the induced character, prove that

  (i) if no element of $\mathcal{C}(g)$ lies in $H$, then $\operatorname{Ind}_{H}^{G}(\psi)(g)=0$,

  (ii) if some element of $\mathcal{C}(g)$ lies in $H$, then

  $$\operatorname{Ind}_{H}^{G}(\psi)(g)=\left|C_{G}(g)\right| \sum_{i=1}^{m} \frac{\psi\left(x_{i}\right)}{\left|C_{H}\left(x_{i}\right)\right|} .$$

  Let $G=S_{4}$ and let $H=\langle a, b\rangle$, where $a=\left(\begin{array}{llll}1 & 2 & 3 & 4\end{array}\right)$ and $b=\left(\begin{array}{l}1\end{array}\right.$ dihedral group and write down its character table. Restrict each $G$-conjugacy class to $H$ and calculate the $H$-conjugacy classes contained in each restriction. Given a character $\psi$ of $H$, express Ind ${ }_{H}^{G}(\psi)(g)$ in terms of $\psi$, where $g$ runs through a set of conjugacy classes of $G$. Use your calculation to find the values of all the irreducible characters of $H$ induced to $G$.

  $$\begin{aligned} & a=\left(\begin{array}{lllll}1 & 2 & 3 & 5 & 6\end{array}\right)(789101112), \\ & b=\left(\begin{array}{llll}1 & 7 & 4 & 10\end{array}\right)\left(\begin{array}{llll}2 & 12 & 5 & 9\end{array}\right)(368), \end{aligned}$$

  comment

• Paper 3, Section II, F

  Representation Theory

  Part II, 2010

  Show that the degree of a complex irreducible character of a finite group is a factor of the order of the group.

  State and prove Burnside's $p^{a} q^{b}$ theorem. You should quote clearly any results you use.

  Prove that for any group of odd order $n$ having precisely $k$ conjugacy classes, the integer $n-k$ is divisible by 16 .

  comment

• Paper 4, Section II, F

  Representation Theory

  Part II, 2010

  Define the circle group $U(1)$. Give a complete list of the irreducible representations of $U(1)$

  Define the spin group $G=S U(2)$, and explain briefly why it is homeomorphic to the unit 3-sphere in $\mathbb{R}^{4}$. Identify the conjugacy classes of $G$ and describe the classification of the irreducible representations of $G$. Identify the characters afforded by the irreducible representations. You need not give detailed proofs but you should define all the terms you use.

  Let $G$ act on the space $\mathrm{M}_{3}(\mathbb{C})$ of $3 \times 3$ complex matrices by conjugation, where $A \in S U(2)$ acts by

  $$A: M \mapsto A_{1} M A_{1}^{-1}$$

  in which $A_{1}$ denotes the $3 \times 3$ block diagonal matrix $\left(\begin{array}{cc}A & 0 \\ 0 & 1\end{array}\right)$. Show that this gives a representation of $G$ and decompose it into irreducibles.

  comment

• Paper 1, Section II, I

  Representation Theory

  Part II, 2011

  Let $G$ be a finite group and $Z$ its centre. Suppose that $G$ has order $n$ and $Z$ has order $m$. Suppose that $\rho: G \rightarrow \mathrm{GL}(V)$ is a complex irreducible representation of degree $d .$

  (i) For $g \in Z$, show that $\rho(g)$ is a scalar multiple of the identity.

  (ii) Deduce that $d^{2} \leqslant n / m$.

  (iii) Show that, if $\rho$ is faithful, then $Z$ is cyclic.

  [Standard results may be quoted without proof, provided they are stated clearly.]

  Now let $G$ be a group of order 18 containing an elementary abelian subgroup $P$ of order 9 and an element $t$ of order 2 with $t x t^{-1}=x^{-1}$ for each $x \in P$. By considering the action of $P$ on an irreducible $\mathbb{C} G$-module prove that $G$ has no faithful irreducible complex representation.

  comment

• Paper 2, Section II, I

  Representation Theory

  Part II, 2011

  State Maschke's Theorem for finite-dimensional complex representations of the finite group $G$. Show by means of an example that the requirement that $G$ be finite is indispensable.

  Now let $G$ be a (possibly infinite) group and let $H$ be a normal subgroup of finite index $r$ in $G$. Let $g_{1}, \ldots, g_{r}$ be representatives of the cosets of $H$ in $G$. Suppose that $V$ is a finite-dimensional completely reducible $\mathbb{C} G$-module. Show that

  (i) if $U$ is a $\mathbb{C} H$-submodule of $V$ and $g \in G$, then the set $g U=\{g u: u \in U\}$ is a $\mathbb{C} H$-submodule of $V$;

  (ii) if $U$ is a $\mathbb{C} H$-submodule of $V$, then $\sum_{i=1}^{r} g_{i} U$ is a $\mathbb{C} G$-submodule of $V$;

  (iii) $V$ is completely reducible regarded as a $\mathbb{C} H$-module.

  Hence deduce that if $\chi$ is an irreducible character of the finite group $G$ then all the constituents of $\chi_{H}$ have the same degree.

  comment

• Paper 3, Section II, I

  Representation Theory

  Part II, 2011

  Define the character $\operatorname{Ind}_{H}^{G} \psi$ of a finite group $G$ which is induced by a character $\psi$ of a subgroup $H$ of $G$.

  State and prove the Frobenius reciprocity formula for the characters $\psi$ of $H$ and $\chi$ of $G$.

  Now suppose that $H$ has index 2 in $G$. An irreducible character $\psi$ of $H$ and an irreducible character $\chi$ of $G$ are said to be 'related' if

  $$\left\langle\operatorname{Ind}_{H}^{G} \psi, \chi\right\rangle_{G}=\left\langle\psi, \operatorname{Res}_{H}^{G} \chi\right\rangle_{H}>0$$

  Show that each $\psi$ of degree $d$ is either 'monogamous' in the sense that it is related to one $\chi$ (of degree $2 d$ ), or 'bigamous' in the sense that it is related to precisely two distinct characters $\chi_{1}, \chi_{2}$ (of degree $\left.d\right)$. Show that each $\chi$ is related to one bigamous $\psi$, or to two monogamous characters $\psi_{1}, \psi_{2}$ (of the same degree).

  Write down the degrees of the complex irreducible characters of the alternating group $A_{5}$. Find the degrees of the irreducible characters of a group $G$ containing $A_{5}$ as a subgroup of index 2 , distinguishing two possible cases.

  comment

• Paper 4, Section II, I

  Representation Theory

  Part II, 2011

  Define the groups $\mathrm{SU}(2)$ and $\mathrm{SO}(3)$.

  Show that $G=\mathrm{SU}(2)$ acts on the vector space of $2 \times 2$ complex matrices of the form

  $$V=\left\{A=\left(\begin{array}{cc} a & b \\ c & -a \end{array}\right) \in \mathrm{M}_{2}(\mathbb{C}): A+\overline{A^{t}}=0\right\}$$

  by conjugation. Denote the corresponding representation of $\mathrm{SU}(2)$ on $V$ by $\rho$.

  Prove the following assertions about this action:

  (i) The subspace $V$ is isomorphic to $\mathbb{R}^{3}$.

  (ii) The pairing $(A, B) \mapsto-\operatorname{tr}(A B)$ defines a positive definite non-degenerate $\mathrm{SU}(2)$ invariant bilinear form.

  (iii) The representation $\rho$ maps $G$ into $\mathrm{SO}(3)$. [You may assume that for any compact group $H$, and any $n \in \mathbb{N}$, there is a continuous group homomorphism $H \rightarrow \mathrm{O}(n)$ if and only if $H$ has an $n$-dimensional representation over $\mathbb{R}$.]

  Write down an orthonormal basis for $V$ and use it to show that $\rho$ is surjective with kernel $\{\pm I\}$.

  Use the isomorphism $\mathrm{SO}(3) \cong G /\{\pm I\}$ to write down a list of irreducible representations of $\mathrm{SO}(3)$ in terms of irreducibles for $\mathrm{SU}(2)$. [Detailed explanations are not required.]

  comment

• Paper 4, Section II, H

  Representation Theory

  Part II, 2012

  Write an essay on the finite-dimensional representations of $\mathrm{SU}(2)$, including a proof of their complete reducibility, and a description of the irreducible representations and the decomposition of their tensor products.

  comment

• Paper 3, Section II, H

  Representation Theory

  Part II, 2012

  Show that every complex representation of a finite group $G$ is equivalent to a unitary representation. Let $\chi$ be a character of some finite group $G$ and let $g \in G$. Explain why there are roots of unity $\omega_{1}, \ldots, \omega_{d}$ such that

  $$\chi\left(g^{i}\right)=\omega_{1}^{i}+\cdots+\omega_{d}^{i}$$

  for all integers $i$.

  For the rest of the question let $G$ be the symmetric group on some finite set. Explain why $\chi(g)=\chi\left(g^{i}\right)$ whenever $i$ is coprime to the order of $g$.

  Prove that $\chi(g) \in \mathbb{Z}$.

  State without proof a formula for $\sum_{g \in G} \chi(g)^{2}$ when $\chi$ is irreducible. Is there an irreducible character $\chi$ of degree at least 2 with $\chi(g) \neq 0$ for all $g \in G$ ? Explain your answer.

  [You may assume basic facts about the symmetric group, and about algebraic integers, without proof. You may also use without proof the fact that $\sum_{\substack{1 \leqslant i \leqslant n \\ \operatorname{gcd}(i, n)=1}} \omega^{i} \in \mathbb{Z}$ for any $n$th root of unity $\omega .]$

  comment

• Paper 2, Section II, H

  Representation Theory

  Part II, 2012

  Suppose that $G$ is a finite group. Define the inner product of two complex-valued class functions on $G$. Prove that the characters of the irreducible representations of $G$ form an orthonormal basis for the space of complex-valued class functions.

  Suppose that $p$ is a prime and $\mathbb{F}_{p}$ is the field of $p$ elements. Let $G=\mathrm{GL}_{2}\left(\mathbb{F}_{p}\right)$. List the conjugacy classes of $G$.

  Let $G$ act naturally on the set of lines in the space $\mathbb{F}_{p}^{2}$. Compute the corresponding permutation character and show that it is reducible. Decompose this character as a sum of two irreducible characters.

  comment

• Paper 1, Section II, 19H

  Representation Theory

  Part II, 2012

  Write down the character table of $D_{10}$.

  Suppose that $G$ is a group of order 60 containing 24 elements of order 5,20 elements of order 3 and 15 elements of order 2 . Calculate the character table of $G$, justifying your answer.

  [You may assume the formula for induction of characters, provided you state it clearly.]

  comment

• Paper 3, Section II, G

  Representation Theory

  Part II, 2013

  Suppose that $\left(\rho_{1}, V_{1}\right)$ and $\left(\rho_{2}, V_{2}\right)$ are complex representations of the finite groups $G_{1}$ and $G_{2}$ respectively. Use $\rho_{1}$ and $\rho_{2}$ to construct a representation $\rho_{1} \otimes \rho_{2}$ of $G_{1} \times G_{2}$ on $V_{1} \otimes V_{2}$ and show that its character satisfies

  $$\chi \rho_{1} \otimes \rho_{2}\left(g_{1}, g_{2}\right)=\chi_{\rho_{1}}\left(g_{1}\right) \chi \rho_{2}\left(g_{2}\right)$$

  for each $g_{1} \in G_{1}, g_{2} \in G_{2}$.

  Prove that if $\rho_{1}$ and $\rho_{2}$ are irreducible then $\rho_{1} \otimes \rho_{2}$ is irreducible as a representation of $G_{1} \times G_{2}$. Moreover, show that every irreducible complex representation of $G_{1} \times G_{2}$ arises in this way.

  Is it true that every complex representation of $G_{1} \times G_{2}$ is of the form $\rho_{1} \otimes \rho_{2}$ with $\rho_{i}$ a complex representation of $G_{i}$ for $i=1,2 ?$ Justify your answer.

  comment

• Paper 2, Section II, G

  Representation Theory

  Part II, 2013

  Recall that a regular icosahedron has 20 faces, 30 edges and 12 vertices. Let $G$ be the group of rotational symmetries of a regular icosahedron.

  Compute the conjugacy classes of $G$. Hence, or otherwise, construct the character table of $G$. Using the character table explain why $G$ must be a simple group.

  [You may use any general theorems provided that you state them clearly.]

  comment

• Paper 4, Section II, G

  Representation Theory

  Part II, 2013

  State and prove Burnside's $p^{a} q^{b}$-theorem.

  comment

• Paper 1, Section II, 19G

  Representation Theory

  Part II, 2013

  State and prove Maschke's Theorem for complex representations of finite groups.

  Without using character theory, show that every irreducible complex representation of the dihedral group of order $10, D_{10}$, has dimension at most two. List the irreducible complex representations of $D_{10}$ up to isomorphism.

  Let $V$ be the set of vertices of a regular pentagon with the usual action of $D_{10}$. Explicitly decompose the permutation representation $\mathbb{C} V$ into a direct sum of irreducible subrepresentations.

  comment

• Paper 4, Section II, H

  Representation Theory

  Part II, 2014

  Let $G=\mathrm{SU}(2)$.

  (i) Sketch a proof that there is an isomorphism of topological groups $G /\{\pm I\} \cong$ $\mathrm{SO}(3)$

  (ii) Let $V_{2}$ be the irreducible complex representation of $G$ of dimension 3. Compute the character of the (symmetric power) representation $S^{n}\left(V_{2}\right)$ of $G$ for any $n \geqslant 0$. Show that the dimension of the space of invariants $\left(S^{n}\left(V_{2}\right)\right)^{G}$, meaning the subspace of $S^{n}\left(V_{2}\right)$ where $G$ acts trivially, is 1 for $n$ even and 0 for $n$ odd. [Hint: You may find it helpful to restrict to the unit circle subgroup $S^{1} \leqslant G$. The irreducible characters of $G$ may be quoted without proof.]

  Using the fact that $V_{2}$ yields the standard 3-dimensional representation of $\mathrm{SO}(3)$, show that $\bigoplus_{n \geqslant 0} S^{n} V_{2} \cong \mathbb{C}[x, y, z]$. Deduce that the ring of complex polynomials in three variables $x, y, z$ which are invariant under the action of $\mathrm{SO}(3)$ is a polynomial ring in one generator. Find a generator for this polynomial ring.

  comment

• Paper 3, Section II, H

  Representation Theory

  Part II, 2014

  (i) State Frobenius' theorem for transitive permutation groups acting on a finite set. Define Frobenius group and show that any finite Frobenius group (with an appropriate action) satisfies the hypotheses of Frobenius' theorem.

  (ii) Consider the group

  $$F_{p, q}:=\left\langle a, b: a^{p}=b^{q}=1, b^{-1} a b=a^{u}\right\rangle$$

  where $p$ is prime, $q$ divides $p-1$ ( $q$ not necessarily prime), and $u$ has multiplicative order $q$ modulo $p$ (such elements $u$ exist since $q$ divides $p-1)$. Let $S$ be the subgroup of $\mathbb{Z}_{p}^{\times}$ consisting of the powers of $u$, so that $|S|=q$. Write $r=(p-1) / q$, and let $v_{1}, \ldots, v_{r}$ be coset representatives for $S$ in $\mathbb{Z}_{p}^{\times}$.

  (a) Show that $F_{p, q}$ has $q+r$ conjugacy classes and that a complete list of the classes comprises $\{1\},\left\{a^{v_{j} s}: s \in S\right\}(1 \leqslant j \leqslant r)$ and $\left\{a^{m} b^{n}: 0 \leqslant m \leqslant p-1\right\}(1 \leqslant n \leqslant q-1)$.

  (b) By observing that the derived subgroup $F_{p, q}^{\prime}=\langle a\rangle$, find $q$ 1-dimensional characters of $F_{p, q}$. [Appropriate results may be quoted without proof.]

  (c) Let $\varepsilon=e^{2 \pi i / p}$. For $v \in \mathbb{Z}_{p}^{\times}$denote by $\psi_{v}$ the character of $\langle a\rangle$ defined by $\psi_{v}\left(a^{x}\right)=\varepsilon^{v x}(0 \leqslant x \leqslant p-1)$. By inducing these characters to $F_{p, q}$, or otherwise, find $r$ distinct irreducible characters of degree $q$.

  comment

• Paper 2, Section II, H

  Representation Theory

  Part II, 2014

  In this question work over $\mathbb{C}$. Let $H$ be a subgroup of $G$. State Mackey's restriction formula, defining all the terms you use. Deduce Mackey's irreducibility criterion.

  Let $G=\left\langle g, r: g^{m}=r^{2}=1, r g r^{-1}=g^{-1}\right\rangle$ (the dihedral group of order $2 m$ ) and let $H=\langle g\rangle$ (the cyclic subgroup of $G$ of order $m$ ). Write down the $m$ inequivalent irreducible characters $\chi_{k}(1 \leqslant k \leqslant m)$ of $H$. Determine the values of $k$ for which the induced character $\operatorname{Ind}_{H}^{G} \chi_{k}$ is irreducible.

  comment

• Paper 1, Section II, H

  Representation Theory

  Part II, 2014

  (i) Let $K$ be any field and let $\lambda \in K$. Let $J_{\lambda, n}$ be the $n \times n$ Jordan block

  $$J_{\lambda, n}=\left(\begin{array}{ccccc} \lambda & 1 & 0 & \cdots & 0 \\ 0 & \ddots & \ddots & & \vdots \\ \vdots & & \ddots & \ddots & 0 \\ \vdots & & & \ddots & 1 \\ 0 & \cdots & \cdots & 0 & \lambda \end{array}\right)$$

  Compute $J_{\lambda, n}^{r}$ for each $r \geqslant 0$.

  (ii) Let $G$ be a cyclic group of order $N$, and let $K$ be an algebraically closed field of characteristic $p \geqslant 0$. Determine all the representations of $G$ on vector spaces over $K$, up to equivalence. Which are irreducible? Which do not split as a direct sum $W \oplus W^{\prime}$, with $W \neq 0$ and $W^{\prime} \neq 0 ?$

  comment

• Paper 4, Section II, F

  Representation Theory

  Part II, 2015

  (a) Let $S^{1}$ be the circle group. Assuming any required facts about continuous functions from real analysis, show that every 1-dimensional continuous representation of $S^{1}$ is of the form

  $$z \mapsto z^{n}$$

  for some $n \in \mathbb{Z}$.

  (b) Let $G=S U(2)$, and let $\rho_{V}$ be a continuous representation of $G$ on a finitedimensional vector space $V$.

  (i) Define the character $\chi_{V}$ of $\rho_{V}$, and show that $\chi_{V} \in \mathbb{N}\left[z, z^{-1}\right]$.

  (ii) Show that $\chi_{V}(z)=\chi_{V}\left(z^{-1}\right)$.

  (iii) Let $V$ be the irreducible 4-dimensional representation of $G$. Decompose $V \otimes V$ into irreducible representations. Hence decompose the exterior square $\Lambda^{2} V$ into irreducible representations.

  comment

• Paper 3, Section II, F

  Representation Theory

  Part II, 2015

  (a) State Mackey's theorem, defining carefully all the terms used in the statement.

  (b) Let $G$ be a finite group and suppose that $G$ acts on the set $\Omega$.

  If $n \in \mathbb{N}$, we say that the action of $G$ on $\Omega$ is $n$-transitive if $\Omega$ has at least $n$ elements and for every pair of $n$-tuples $\left(a_{1}, \ldots, a_{n}\right)$ and $\left(b_{1}, \ldots, b_{n}\right)$ such that the $a_{i}$ are distinct elements of $\Omega$ and the $b_{i}$ are distinct elements of $\Omega$, there exists $g \in G$ with $g a_{i}=b_{i}$ for every $i$.

  (i) Let $\Omega$ have at least $n$ elements, where $n \geqslant 1$ and let $\omega \in \Omega$. Show that $G$ acts $n$-transitively on $\Omega$ if and only if $G$ acts transitively on $\Omega$ and the stabiliser $G_{\omega}$ acts $(n-1)$-transitively on $\Omega \backslash\{\omega\}$.

  (ii) Show that the permutation module $\mathbb{C} \Omega$ can be decomposed as

  $$\mathbb{C} \Omega=\mathbb{C}_{G} \oplus V,$$

  where $\mathbb{C}_{G}$ is the trivial module and $V$ is some $\mathbb{C} G$-module.

  (iii) Assume that $|\Omega| \geqslant 2$, so that $V \neq 0$. Prove that $V$ is irreducible if and only if $G$ acts 2-transitively on $\Omega$. In that case show also that $V$ is not the trivial representation. [Hint: Pick any orbit of $G$ on $\Omega$; it is isomorphic as a $G$-set to $G / H$ for some subgroup $H \leqslant G$. Consider the induced character $\left.\operatorname{Ind}_{H}^{G} 1_{H} \cdot\right]$

  comment

• Paper 2, Section II, $15 F$

  Representation Theory

  Part II, 2015

  Let $G$ be a finite group. Suppose that $\rho: G \rightarrow G \mathrm{G}(V)$ is a finite-dimensional complex representation of dimension $d$. Let $n \in \mathbb{N}$ be arbitrary.

  (i) Define the $n$th symmetric power $S^{n} V$ and the $n$th exterior power $\Lambda^{n} V$ and write down their respective dimensions.

  Let $g \in G$ and let $\lambda_{1}, \ldots, \lambda_{d}$ be the eigenvalues of $g$ on $V$. What are the eigenvalues of $g$ on $S^{n} V$ and on $\Lambda^{n} V$ ?

  (ii) Let $X$ be an indeterminate. For any $g \in G$, define the characteristic polynomial $Q=Q(g, X)$ of $g$ on $V$ by $Q(g, X):=\operatorname{det}(g-X I)$. What is the relationship between the coefficients of $Q$ and the character $\chi_{\Lambda^{n}} V$ of the exterior power?

  Find a relation between the character $\chi_{S^{n}} V$ of the symmetric power and the polynomial $Q$.

  comment

• Paper 1, Section II, F

  Representation Theory

  Part II, 2015

  (a) Let $G$ be a finite group and let $\rho: G \rightarrow \mathrm{GL}_{2}(\mathbb{C})$ be a representation of $G$. Suppose that there are elements $g, h$ in $G$ such that the matrices $\rho(g)$ and $\rho(h)$ do not commute. Use Maschke's theorem to prove that $\rho$ is irreducible.

  (b) Let $n$ be a positive integer. You are given that the dicyclic group

  $$G_{4 n}=\left\langle a, b: a^{2 n}=1, a^{n}=b^{2}, b^{-1} a b=a^{-1}\right\rangle$$

  has order $4 n$.

  (i) Show that if $\epsilon$ is any $(2 n)$ th root of unity in $\mathbb{C}$, then there is a representation of $G_{4 n}$ over $\mathbb{C}$ which sends

  $$a \mapsto\left(\begin{array}{cc} \epsilon & 0 \\ 0 & \epsilon^{-1} \end{array}\right), \quad b \mapsto\left(\begin{array}{cc} 0 & 1 \\ \epsilon^{n} & 0 \end{array}\right)$$

  (ii) Find all the irreducible representations of $G_{4 n}$.

  (iii) Find the character table of $G_{4 n}$.

  [Hint: You may find it helpful to consider the cases $n$ odd and $n$ even separately.]

  comment

• Paper 3, Section II, I

  Representation Theory

  Part II, 2016

  (a) Let the finite group $G$ act on a finite set $X$ and let $\pi$ be the permutation character. If $G$ is 2 -transitive on $X$, show that $\pi=1_{G}+\chi$, where $\chi$ is an irreducible character of $G$.

  (b) Let $n \geqslant 4$, and let $G$ be the symmetric group $S_{n}$ acting naturally on the set $X=\{1, \ldots, n\}$. For any integer $r \leqslant n / 2$, write $X_{r}$ for the set of all $r$-element subsets of $X$, and let $\pi_{r}$ be the permutation character of the action of $G$ on $X_{r}$. Compute the degree of $\pi_{r}$. If $0 \leqslant \ell \leqslant k \leqslant n / 2$, compute the character inner product $\left\langle\pi_{k}, \pi_{\ell}\right\rangle$.

  Let $m=n / 2$ if $n$ is even, and $m=(n-1) / 2$ if $n$ is odd. Deduce that $S_{n}$ has distinct irreducible characters $\chi^{(n)}=1_{G}, \chi^{(n-1,1)}, \chi^{(n-2,2)}, \ldots, \chi^{(n-m, m)}$ such that for all $r \leqslant m$,

  $$\pi_{r}=\chi^{(n)}+\chi^{(n-1,1)}+\chi^{(n-2,2)}+\cdots+\chi^{(n-r, r)}$$

  (c) Let $\Omega$ be the set of all ordered pairs $(i, j)$ with $i, j \in\{1,2, \ldots, n\}$ and $i \neq j$. Let $S_{n}$ act on $\Omega$ in the obvious way. Write $\pi^{(n-2,1,1)}$ for the permutation character of $S_{n}$ in this action. By considering inner products, or otherwise, prove that

  $$\pi^{(n-2,1,1)}=1+2 \chi^{(n-1,1)}+\chi^{(n-2,2)}+\psi$$

  where $\psi$ is an irreducible character. Calculate the degree of $\psi$, and calculate its value on the elements $\left(\begin{array}{ll}1 & 2\end{array}\right)$ and $\left(\begin{array}{lll}1 & 2 & 3)\end{array}\right)$ of $S_{n}$.

  comment

• Paper 2, Section II, I

  Representation Theory

  Part II, 2016

  Show that the 1-dimensional (complex) characters of a finite group $G$ form a group under pointwise multiplication. Denote this group by $\widehat{G}$. Show that if $g \in G$, the map $\chi \mapsto \chi(g)$ from $\widehat{G}$to $\mathbb{C}$ is a character of $\widehat{G}$, hence an element of $\widehat{G}$. What is the kernel of the $\operatorname{map} G \rightarrow \widehat{\widehat{G}}$?

  Show that if $G$ is abelian the map $G \rightarrow \widehat{\widehat{G}}$is an isomorphism. Deduce, from the structure theorem for finite abelian groups, that the groups $G$ and $\widehat{G}$are isomorphic as abstract groups.

  comment

• Paper 4, Section II, I

  Representation Theory

  Part II, 2016

  Let $N$ be a proper normal subgroup of a finite group $G$ and let $U$ be an irreducible complex representation of $G$. Show that either $U$ restricted to $N$ is a sum of copies of a single irreducible representation of $N$, or else $U$ is induced from an irreducible representation of some proper subgroup of $G$.

  Recall that a $p$-group is a group whose order is a power of the prime number $p$. Deduce, by induction on the order of the group, or otherwise, that every irreducible complex representation of a $p$-group is induced from a 1-dimensional representation of some subgroup.

  [You may assume that a non-abelian $p$-group $G$ has an abelian normal subgroup which is not contained in the centre of $G$.]

  comment

• Paper 1, Section II, I

  Representation Theory

  Part II, 2016

  Let $N$ be a normal subgroup of the finite group $G$. Explain how a (complex) representation of $G / N$ gives rise to an associated representation of $G$, and briefly describe which representations of $G$ arise this way.

  Let $G$ be the group of order 54 which is given by

  $$G=\left\langle a, b: a^{9}=b^{6}=1, b^{-1} a b=a^{2}\right\rangle$$

  Find the conjugacy classes of $G$. By observing that $N_{1}=\langle a\rangle$ and $N_{2}=\left\langle a^{3}, b^{2}\right\rangle$ are normal in $G$, or otherwise, construct the character table of $G$.

  comment

• Paper 2, Section II, G

  Representation Theory

  Part II, 2017

  In this question you may assume the following result. Let $\chi$ be a character of a finite group $G$ and let $g \in G$. If $\chi(g)$ is a rational number, then $\chi(g)$ is an integer.

  (a) If $a$ and $b$ are positive integers, we denote their highest common factor by $(a, b)$. Let $g$ be an element of order $n$ in the finite group $G$. Suppose that $g$ is conjugate to $g^{i}$ for all $i$ with $1 \leqslant i \leqslant n$ and $(i, n)=1$. Prove that $\chi(g)$ is an integer for all characters $\chi$ of $G$.

  [You may use the following result without proof. Let $\omega$ be an $n$th root of unity. Then

  is an integer.]

  Deduce that all the character values of symmetric groups are integers.

  (b) Let $G$ be a group of odd order.

  Let $\chi$ be an irreducible character of $G$ with $\chi=\bar{\chi}$. Prove that

  $$\left\langle\chi, 1_{G}\right\rangle=\frac{1}{|G|}(\chi(1)+2 \alpha),$$

  where $\alpha$ is an algebraic integer. Deduce that $\chi=1_{G}$.

  $$\begin{aligned} & \sum_{1 \leqslant i \leqslant n,} \omega^{i} \\ & \begin{aligned}&1 \leqslant i \leqslant n \\&(i, n)=1\end{aligned} \end{aligned}$$

  comment

• Paper 3, Section II, G

  Representation Theory

  Part II, 2017

  (a) State Burnside's $p^{a} q^{b}$ theorem.

  (b) Let $P$ be a non-trivial group of prime power order. Show that if $H$ is a non-trivial normal subgroup of $P$, then $H \cap Z(P) \neq\{1\}$.

  Deduce that a non-abelian simple group cannot have an abelian subgroup of prime power index.

  (c) Let $\rho$ be a representation of the finite group $G$ over $\mathbb{C}$. Show that $\delta: g \mapsto \operatorname{det}(\rho(g))$ is a linear character of $G$. Assume that $\delta(g)=-1$ for some $g \in G$. Show that $G$ has a normal subgroup of index 2 .

  Now let $E$ be a group of order $2 k$, where $k$ is an odd integer. By considering the regular representation of $E$, or otherwise, show that $E$ has a normal subgroup of index $2 .$

  Deduce that if $H$ is a non-abelian simple group of order less than 80 , then $H$ has order 60 .

  comment

• Paper 1, Section II, G

  Representation Theory

  Part II, 2017

  (a) Prove that if there exists a faithful irreducible complex representation of a finite group $G$, then the centre $Z(G)$ is cyclic.

  (b) Define the permutations $a, b, c \in S_{6}$ by

  $$a=\left(\begin{array}{lll} 1 & 2 & 3 \end{array}\right), b=\left(\begin{array}{lll} 4 & 5 & 6 \end{array}\right), c=\left(\begin{array}{ll} 2 & 3 \end{array}\right)(45),$$

  and let $E=\langle a, b, c\rangle$.

  (i) Using the relations $a^{3}=b^{3}=c^{2}=1, a b=b a, c^{-1} a c=a^{-1}$ and $c^{-1} b c=b^{-1}$, prove that $E$ has order 18 .

  (ii) Suppose that $\varepsilon$ and $\eta$ are complex cube roots of unity. Prove that there is a (matrix) representation $\rho$ of $E$ over $\mathbb{C}$ such that

  $$a \mapsto\left(\begin{array}{cc} \varepsilon & 0 \\ 0 & \varepsilon^{-1} \end{array}\right), \quad b \mapsto\left(\begin{array}{cc} \eta & 0 \\ 0 & \eta^{-1} \end{array}\right), \quad c \mapsto\left(\begin{array}{ll} 0 & 1 \\ 1 & 0 \end{array}\right)$$

  (iii) For which values of $\varepsilon, \eta$ is $\rho$ faithful? For which values of $\varepsilon, \eta$ is $\rho$ irreducible?

  (c) Note that $\langle a, b\rangle$ is a normal subgroup of $E$ which is isomorphic to $C_{3} \times C_{3}$. By inducing linear characters of this subgroup, or otherwise, obtain the character table of $E$.

  Deduce that $E$ has the property that $Z(E)$ is cyclic but $E$ has no faithful irreducible representation over $\mathbb{C}$.

  comment

• Paper 4, Section II, G

  Representation Theory

  Part II, 2017

  Let $G=\mathrm{SU}(2)$ and let $V_{n}$ be the vector space of complex homogeneous polynomials of degree $n$ in two variables.

  (a) Prove that $V_{n}$ has the structure of an irreducible representation for $G$.

  (b) State and prove the Clebsch-Gordan theorem.

  (c) Quoting without proof any properties of symmetric and exterior powers which you need, decompose $\mathrm{S}^{2} V_{n}$ and $\Lambda^{2} V_{n}(n \geqslant 1)$ into irreducible $G$-spaces.

  comment

• Paper 1, Section II, I

  Representation Theory

  Part II, 2018

  (a) Define the derived subgroup, $G^{\prime}$, of a finite group $G$. Show that if $\chi$ is a linear character of $G$, then $G^{\prime} \leqslant$ ker $\chi$. Prove that the linear characters of $G$ are precisely the lifts to $G$ of the irreducible characters of $G / G^{\prime}$. [You should state clearly any additional results that you require.]

  (b) For $n \geqslant 1$, you may take as given that the group

  $$G_{6 n}:=\left\langle a, b: a^{2 n}=b^{3}=1, a^{-1} b a=b^{-1}\right\rangle$$

  has order $6 n$.

  (i) Let $\omega=e^{2 \pi i / 3}$. Show that if $\varepsilon$ is any $(2 n)$-th root of unity in $\mathbb{C}$, then there is a representation of $G_{6 n}$ over $\mathbb{C}$ which sends

  $$a \mapsto\left(\begin{array}{ll} 0 & \varepsilon \\ \varepsilon & 0 \end{array}\right), \quad b \mapsto\left(\begin{array}{cc} \omega & 0 \\ 0 & \omega^{2} \end{array}\right)$$

  (ii) Find all the irreducible representations of $G_{6 n}$.

  (iii) Find the character table of $G_{6 n}$.

  comment

• Paper 2, Section II, I

  Representation Theory

  Part II, 2018

  (a) Suppose $H$ is a subgroup of a finite group $G, \chi$ is an irreducible character of $G$ and $\varphi_{1}, \ldots, \varphi_{r}$ are the irreducible characters of $H$. Show that in the restriction $\chi \downarrow_{H}=a_{1} \varphi_{1}+\cdots+a_{r} \varphi_{r}$, the multiplicities $a_{1}, \ldots, a_{r}$ satisfy

  $$\sum_{i=1}^{r} a_{i}^{2} \leqslant|G: H|$$

  Determine necessary and sufficient conditions under which the inequality in ( $\uparrow$ ) is actually an equality.

  (b) Henceforth suppose that $H$ is a (normal) subgroup of index 2 in $G$, and that $\chi$ is an irreducible character of $G$.

  Lift the non-trivial linear character of $G / H$ to obtain a linear character of $G$ which satisfies

  $$\lambda(g)= \begin{cases}1 & \text { if } g \in H \\ -1 & \text { if } g \notin H\end{cases}$$

  (i) Show that the following are equivalent:

  (1) $\chi \downarrow_{H}$ is irreducible;

  (2) $\chi(g) \neq 0$ for some $g \in G$ with $g \notin H$;

  (3) the characters $\chi$ and $\chi \lambda$ of $G$ are not equal.

  (ii) Suppose now that $\chi \downarrow_{H}$ is irreducible. Show that if $\psi$ is an irreducible character of $G$ which satisfies

  $$\psi \downarrow_{H}=\chi \downarrow_{H}$$

  then either $\psi=\chi$ or $\psi=\chi \lambda .$

  (iii) Suppose that $\chi \downarrow_{H}$ is the sum of two irreducible characters of $H$, say $\chi \downarrow_{H}=\psi_{1}+\psi_{2}$. If $\phi$ is an irreducible character of $G$ such that $\phi \downarrow_{H}$ has $\psi_{1}$ or $\psi_{2}$ as a constituent, show that $\phi=\chi$.

  (c) Suppose that $G$ is a finite group with a subgroup $K$ of index 3 , and let $\chi$ be an irreducible character of $G$. Prove that

  $$\left\langle\chi \downarrow_{K}, \chi \downarrow_{K}\right\rangle_{K}=1,2 \text { or } 3$$

  Give examples to show that each possibility can occur, giving brief justification in each case.

  comment

• Paper 3, Section II, I

  Representation Theory

  Part II, 2018

  State the row orthogonality relations. Prove that if $\chi$ is an irreducible character of the finite group $G$, then $\chi(1)$ divides the order of $G$.

  Stating clearly any additional results you use, deduce the following statements:

  (i) Groups of order $p^{2}$, where $p$ is prime, are abelian.

  (ii) If $G$ is a group of order $2 p$, where $p$ is prime, then either the degrees of the irreducible characters of $G$ are all 1 , or they are

  $$1,1,2, \ldots, 2(\text { with }(p-1) / 2 \text { of degree } 2)$$

  (iii) No simple group has an irreducible character of degree 2 .

  (iv) Let $p$ and $q$ be prime numbers with $p>q$, and let $G$ be a non-abelian group of order $p q$. Then $q$ divides $p-1$ and $G$ has $q+((p-1) / q)$ conjugacy classes.

  comment

• Paper 4, Section II, I

  Representation Theory

  Part II, 2018

  Define $G=\mathrm{SU}(2)$ and write down a complete list

  $$\left\{V_{n}: n=0,1,2, \ldots\right\}$$

  of its continuous finite-dimensional irreducible representations. You should define all the terms you use but proofs are not required. Find the character $\chi_{V_{n}}$ of $V_{n}$. State the Clebsch-Gordan formula.

  (a) Stating clearly any properties of symmetric powers that you need, decompose the following spaces into irreducible representations of $G$ :

  (i) $V_{4} \otimes V_{3}, V_{3} \otimes V_{3}, S^{2} V_{3}$;

  (ii) $V_{1} \otimes \cdots \otimes V_{1}$ (with $n$ multiplicands);

  (iii) $S^{3} V_{2}$.

  (b) Let $G$ act on the space $M_{3}(\mathbb{C})$ of $3 \times 3$ complex matrices by

  $$A: X \mapsto A_{1} X A_{1}^{-1}$$

  where $A_{1}$ is the block matrix $\left(\begin{array}{cc}A & 0 \\ 0 & 1\end{array}\right)$. Show that this gives a representation of $G$ and decompose it into irreducible summands.

  comment

• Paper 3, Section II, I

  Representation Theory

  Part II, 2019

  In this question all representations are complex and $G$ is a finite group.

  (a) State and prove Mackey's theorem. State the Frobenius reciprocity theorem.

  (b) Let $X$ be a finite $G$-set and let $\mathbb{C} X$ be the corresponding permutation representation. Pick any orbit of $G$ on $X$ : it is isomorphic as a $G$-set to $G / H$ for some subgroup $H$ of $G$. Write down the character of $\mathbb{C}(G / H)$.

  (i) Let $\mathbb{C}_{G}$ be the trivial representation of $G$. Show that $\mathbb{C} X$ may be written as a direct sum

  $$\mathbb{C} X=\mathbb{C}_{G} \oplus V$$

  for some representation $V$.

  (ii) Using the results of (a) compute the character inner product $\left\langle 1_{H} \uparrow^{G}, 1_{H} \uparrow^{G}\right\rangle_{G}$ in terms of the number of $(H, H)$ double cosets.

  (iii) Now suppose that $|X| \geqslant 2$, so that $V \neq 0$. By writing $\mathbb{C}(G / H)$ as a direct sum of irreducible representations, deduce from (ii) that the representation $V$ is irreducible if and only if $G$ acts 2 -transitively. In that case, show that $V$ is not the trivial representation.

  comment

• Paper 4, Section II, I

  Representation Theory

  Part II, 2019

  (a) What is meant by a compact topological group? Explain why $\mathrm{SU}(n)$ is an example of such a group.

  [In the following the existence of a Haar measure for any compact Hausdorff topological group may be assumed, if required.]

  (b) Let $G$ be any compact Hausdorff topological group. Show that there is a continuous group homomorphism $\rho: G \rightarrow \mathrm{O}(n)$ if and only if $G$ has an $n$-dimensional representation over $\mathbb{R}$. [Here $\mathrm{O}(n)$ denotes the subgroup of $\mathrm{GL}_{n}(\mathbb{R})$ preserving the standard (positive-definite) symmetric bilinear form.]

  (c) Explicitly construct such a representation $\rho: \mathrm{SU}(2) \rightarrow \mathrm{SO}(3)$ by showing that $\mathrm{SU}(2)$ acts on the following vector space of matrices,

  $$\left\{A=\left(\begin{array}{cc} a & b \\ c & -a \end{array}\right) \in \mathrm{M}_{2}(\mathbb{C}): A+\overline{A^{t}}=0\right\}$$

  by conjugation.

  Show that

  (i) this subspace is isomorphic to $\mathbb{R}^{3}$;

  (ii) the trace map $(A, B) \mapsto-\operatorname{tr}(A B)$ induces an invariant positive definite symmetric bilinear form;

  (iii) $\rho$ is surjective with kernel $\left\{\pm I_{2}\right\}$. [You may assume, without proof, that $\mathrm{SU}(2)$ is connected.]

  comment

• Paper 2, Section II, I

  Representation Theory

  Part II, 2019

  (a) For any finite group $G$, let $\rho_{1}, \ldots, \rho_{k}$ be a complete set of non-isomorphic complex irreducible representations of $G$, with dimensions $n_{1}, \ldots n_{k}$, respectively. Show that

  $$\sum_{j=1}^{k} n_{j}^{2}=|G|$$

  (b) Let $A, B, C, D$ be the matrices

  and let $G=\langle A, B, C, D\rangle$. Write $Z=-I_{4}$.

  (i) Prove that the derived subgroup $G^{\prime}=\langle Z\rangle$.

  (ii) Show that for all $g \in G, g^{2} \in\langle Z\rangle$, and deduce that $G$ is a 2-group of order at most 32 .

  (iii) Prove that the given representation of $G$ of degree 4 is irreducible.

  (iv) Prove that $G$ has order 32 , and find all the irreducible representations of $G$.

  $$\begin{aligned} & A=\left(\begin{array}{cccc}1 & 0 & 0 & 0 \\0 & -1 & 0 & 0 \\0 & 0 & 1 & 0 \\0 & 0 & 0 & -1\end{array}\right), \quad B=\left(\begin{array}{llll}0 & 1 & 0 & 0 \\1 & 0 & 0 & 0 \\0 & 0 & 0 & 1 \\0 & 0 & 1 & 0\end{array}\right) \\ & C=\left(\begin{array}{cccc}1 & 0 & 0 & 0 \\0 & 1 & 0 & 0 \\0 & 0 & -1 & 0 \\0 & 0 & 0 & -1\end{array}\right), \quad D=\left(\begin{array}{llll}0 & 0 & 1 & 0 \\0 & 0 & 0 & 1 \\1 & 0 & 0 & 0 \\0 & 1 & 0 & 0\end{array}\right) \end{aligned}$$

  comment

• Paper 1, Section II, I

  Representation Theory

  Part II, 2019

  (a) State and prove Schur's lemma over $\mathbb{C}$.

  In the remainder of this question we work over $\mathbb{R}$.

  (b) Let $G$ be the cyclic group of order 3 .

  (i) Write the regular $\mathbb{R} G$-module as a direct sum of irreducible submodules.

  (ii) Find all the intertwining homomorphisms between the irreducible $\mathbb{R} G$-modules. Deduce that the conclusion of Schur's lemma is false if we replace $\mathbb{C}$ by $\mathbb{R}$.

  (c) Henceforth let $G$ be a cyclic group of order $n$. Show that

  (i) if $n$ is even, the regular $\mathbb{R} G$-module is a direct sum of two (non-isomorphic) 1dimensional irreducible submodules and $(n-2) / 2$ (non-isomorphic) 2-dimensional irreducible submodules;

  (ii) if $n$ is odd, the regular $\mathbb{R} G$-module is a direct sum of one 1-dimensional irreducible submodule and $(n-1) / 2$ (non-isomorphic) 2-dimensional irreducible submodules.

  comment

• Paper 1, Section II, F

  Representation Theory

  Part II, 2020

  State and prove Maschke's theorem.

  Let $G$ be the group of isometries of $\mathbb{Z}$. Recall that $G$ is generated by the elements $t, s$ where $t(n)=n+1$ and $s(n)=-n$ for $n \in \mathbb{Z}$.

  Show that every non-faithful finite-dimensional complex representation of $G$ is a direct sum of subrepresentations of dimension at most two.

  Write down a finite-dimensional complex representation of the group $(\mathbb{Z},+)$ that is not a direct sum of one-dimensional subrepresentations. Hence, or otherwise, find a finitedimensional complex representation of $G$ that is not a direct sum of subrepresentations of dimension at most two. Briefly justify your answer.

  [Hint: You may assume that any non-trivial normal subgroup of $G$ contains an element of the form $t^{m}$ for some $m>0$.]

  comment

• Paper 2, Section II, F

  Representation Theory

  Part II, 2020

  Let $G$ be the unique non-abelian group of order 21 up to isomorphism. Compute the character table of $G$.

  [You may find it helpful to think of $G$ as the group of $2 \times 2$ matrices of the form $\left(\begin{array}{cc}a & b \\ 0 & a^{-1}\end{array}\right)$ with $a, b \in \mathbb{F}_{7}$ and $a^{3}=1$. You may use any standard results from the course provided you state them clearly.]

  comment

• Paper 3, Section II, F

  Representation Theory

  Part II, 2020

  State Mackey's restriction formula and Frobenius reciprocity for characters. Deduce Mackey's irreducibility criterion for an induced representation.

  For $n \geqslant 2$ show that if $S_{n-1}$ is the subgroup of $S_{n}$ consisting of the elements that fix $n$, and $W$ is a complex representation of $S_{n-1}$, then $\operatorname{Ind}_{S_{n-1}}^{S_{n}} W$ is not irreducible.

  comment

• Paper 4, Section II, F

  Representation Theory

  Part II, 2020

  (a) State and prove Burnside's lemma. Deduce that if a finite group $G$ acts 2transitively on a set $X$ then the corresponding permutation character has precisely two (distinct) irreducible summands.

  (b) Suppose that $\mathbb{F}_{q}$ is a field with $q$ elements. Write down a list of conjugacy class representatives for $G L_{2}\left(\mathbb{F}_{q}\right)$. Consider the natural action of $G L_{2}\left(\mathbb{F}_{q}\right)$ on the set of lines through the origin in $\mathbb{F}_{q}^{2}$. What values does the corresponding permutation character take on each conjugacy class representative in your list? Decompose this permutation character into irreducible characters.

  comment

• Paper 1, Section II, I

  Representation Theory

  Part II, 2021

  (a) What does it mean to say that a representation of a group is completely reducible? State Maschke's theorem for representations of finite groups over fields of characteristic 0 . State and prove Schur's lemma. Deduce that if there exists a faithful irreducible complex representation of $G$, then $Z(G)$ is cyclic.

  (b) If $G$ is any finite group, show that the regular representation $\mathbb{C} G$ is faithful. Show further that for every finite simple group $G$, there exists a faithful irreducible complex representation of $G$.

  (c) Which of the following groups have a faithful irreducible representation? Give brief justification of your answers.

  (i) the cyclic groups $C_{n}(n$ a positive integer $)$;

  (ii) the dihedral group $D_{8}$;

  (iii) the direct product $C_{2} \times D_{8}$.

  comment

• Paper 2, Section II, I

  Representation Theory

  Part II, 2021

  Let $G$ be a finite group and work over $\mathbb{C}$.

  (a) Let $\chi$ be a faithful character of $G$, and suppose that $\chi(g)$ takes precisely $r$ different values as $g$ varies over all the elements of $G$. Show that every irreducible character of $G$ is a constituent of one of the powers $\chi^{0}, \chi^{1}, \ldots, \chi^{r-1}$. [Standard properties of the Vandermonde matrix may be assumed if stated correctly.]

  (b) Assuming that the number of irreducible characters of $G$ is equal to the number of conjugacy classes of $G$, show that the irreducible characters of $G$ form a basis of the complex vector space of all class functions on $G$. Deduce that $g, h \in G$ are conjugate if and only if $\chi(g)=\chi(h)$ for all characters $\chi$ of $G$.

  (c) Let $\chi$ be a character of $G$ which is not faithful. Show that there is some irreducible character $\psi$ of $G$ such that $\left\langle\chi^{n}, \psi\right\rangle=0$ for all integers $n \geqslant 0$.

  comment

• Paper 3, Section II, I

  Representation Theory

  Part II, 2021

  In this question we work over $\mathbb{C}$.

  (a) (i) Let $H$ be a subgroup of a finite group $G$. Given an $H$-space $W$, define the complex vector space $V=\operatorname{Ind}_{H}^{G}(W)$. Define, with justification, the $G$-action on $V$.

  (ii) Write $\mathcal{C}(g)$ for the conjugacy class of $g \in G$. Suppose that $H \cap \mathcal{C}(g)$ breaks up into $s$ conjugacy classes of $H$ with representatives $x_{1}, \ldots, x_{s}$. If $\psi$ is a character of $H$, write down, without proof, a formula for the induced character $\operatorname{Ind}_{H}^{G}(\psi)$ as a certain sum of character values $\psi\left(x_{i}\right)$.

  (b) Define permutations $a, b \in S_{7}$ by $a=\left(\begin{array}{llllll}1 & 2 & 3 & 4 & 5 & 6\end{array}\right), b=(235)(476)$ and let $G$ be the subgroup $\langle a, b\rangle$ of $S_{7}$. It is given that the elements of $G$ are all of the form $a^{i} b^{j}$ for $0 \leqslant i \leqslant 6,0 \leqslant j \leqslant 2$ and that $G$ has order 21 .

  (i) Find the orders of the centralisers $C_{G}(a)$ and $C_{G}(b)$. Hence show that there are five conjugacy classes of $G$.

  (ii) Find all characters of degree 1 of $G$ by lifting from a suitable quotient group.

  (iii) Let $H=\langle a\rangle$. By first inducing linear characters of $H$ using the formula stated in part (a)(ii), find the remaining irreducible characters of $G$.

  comment

• Paper 4, Section II, I

  Representation Theory

  Part II, 2021

  (a) Define the group $S^{1}$. Sketch a proof of the classification of the irreducible continuous representations of $S^{1}$. Show directly that the characters obey an orthogonality relation.

  (b) Define the group $S U(2)$.

  (i) Show that there is a bijection between the conjugacy classes in $G=S U(2)$ and the subset $[-1,1]$ of the real line. [If you use facts about a maximal torus $T$, you should prove them.]

  (ii) Write $\mathcal{O}_{x}$ for the conjugacy class indexed by an element $x$, where $-1<x<1$. Show that $\mathcal{O}_{x}$ is homeomorphic to $S^{2}$. [Hint: First show that $\mathcal{O}_{x}$ is in bijection with $G / T$.

  (iii) Let $t: G \rightarrow[-1,1]$ be the parametrisation of conjugacy classes from part (i). Determine the representation of $G$ whose character is the function $g \mapsto 8 t(g)^{3}$.

  comment